home
>
publications
> Acorn User
Acorn
User
is a publication dedicated to
Acorn
's
family of microcomputers: initially the Atom, then the famous BBC
microcomputer, followed by the powerful Acorn Archimedes, then the RISC-PC
(and now ...?).
I bought my first BBC micro in 1983, and was fascinated by the
spectacular fractal effects
that I could generate with very short programs. And programs that short
are ideal for publishing in popular computing magazines!
1991
Susan Stepney. Prime Candidates.
Acorn User
, June 1991.
|
|
Finding
Mersenne primes
,
using the
Lucas-Lehmer test
.
scanned article
(jpeg):
p1
|
p2
|
p3
I state in the article that "
The biggest
[Mersenne prime] known to date [1991] is M
216091
".
Ones a
lot
larger than that
are known nowadays
!
|

|
1988
Susan Stepney. Life on the Line.
Acorn User
, November 1988.
|
|
Conway's well-known 'Game of Life' is a two-dimensional cellular
automata. Here I explore the just-as-complex one-dimensional cellular
automata, the advantage being that you can display multiple
generations simultaneously.
scanned article
(jpeg):
p1
|
p2
|
p3
|
p4
I state in the article that "
It's
probably the case that some of the 1D rules also give
computationally irreducible automata, but this has yet to be proved.
"
It has now been proved for rule 110 (by Cook in 2004) ... and, in a
wonderful coincidence, the listing for this program was
printed on p.110
!
|

|
1984
Susan Stepney. Snowflakes and other monsters.
Acorn User
,
March 1984.
|
|
How to draw 'pathological' fractal curves, by defining the
'generator' shape, and letting the computer iterate. One such 'monster
curve' is the Koch snowflake, which has infinite length, but encloses
a finite area.
scanned article
(jpeg):
p1
|
p2
|
p3
|
p4
|
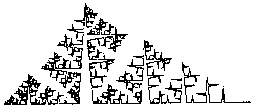
|
1983
Susan Stepney. Incredible fractals.
Acorn User
, October
1983.
|
|
One of the earliest articles on fractals in a popular computing
magazine. Factoid: I had no access to a printer at the time, except
one on an IBM 3081 mainframe. So I had to recode the algorithms in
Fortran to produce the pictures!
scanned article
(jpeg):
p1
|
p2
|
p3
|
p4
|

|